Outstanding Tips About What Is The Curving Graph Called Excel Vba Chart Axes Properties

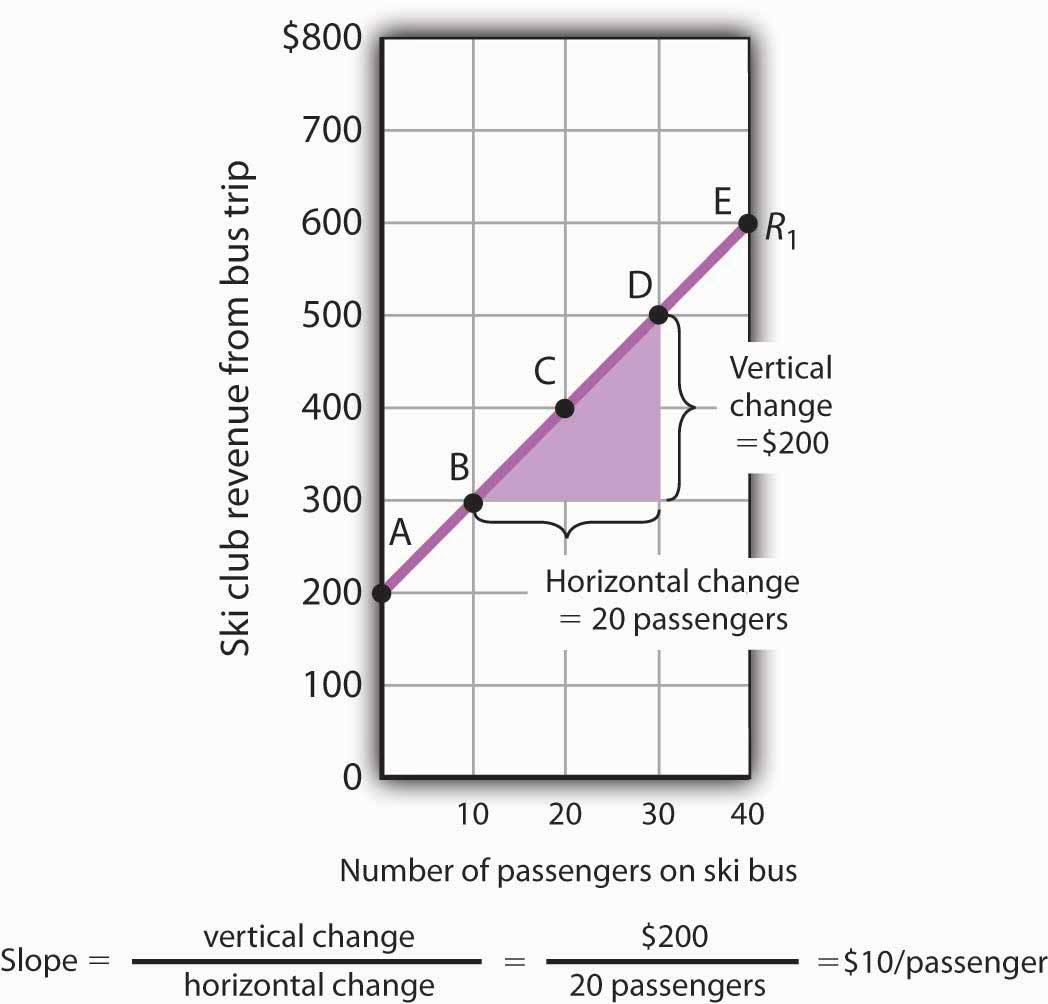
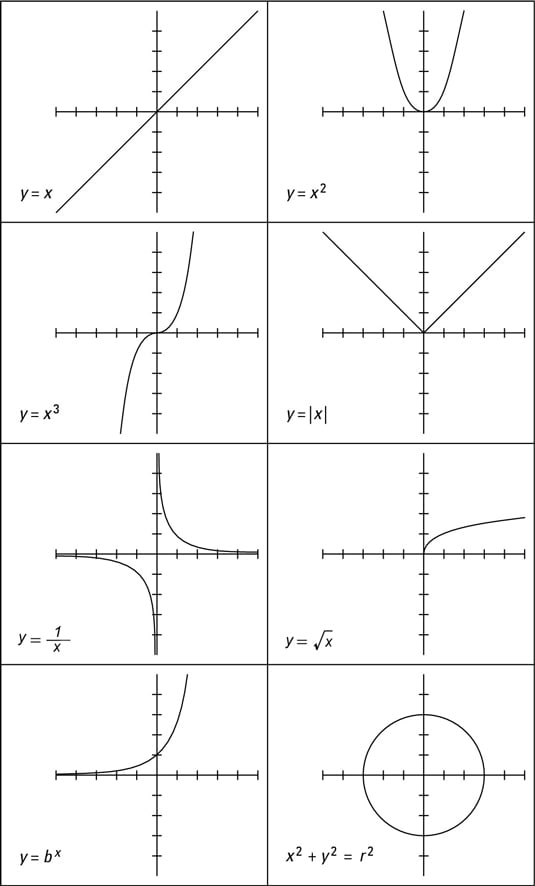
How do the curves differ from each other?

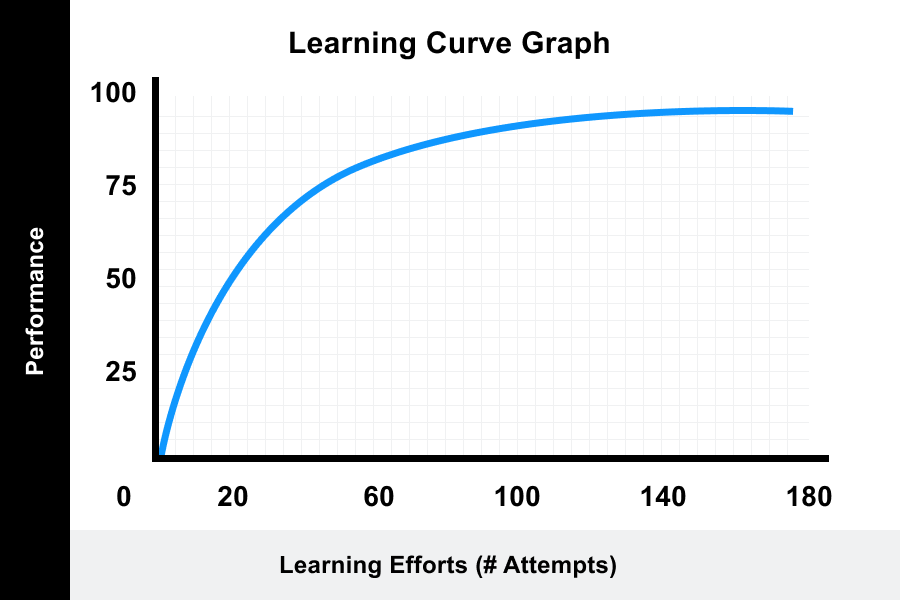
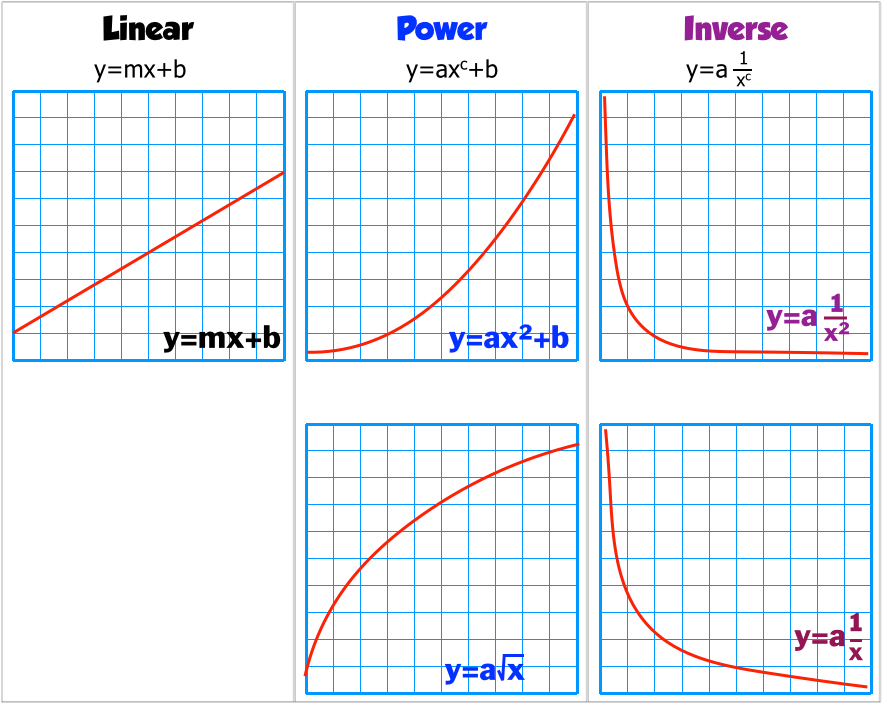
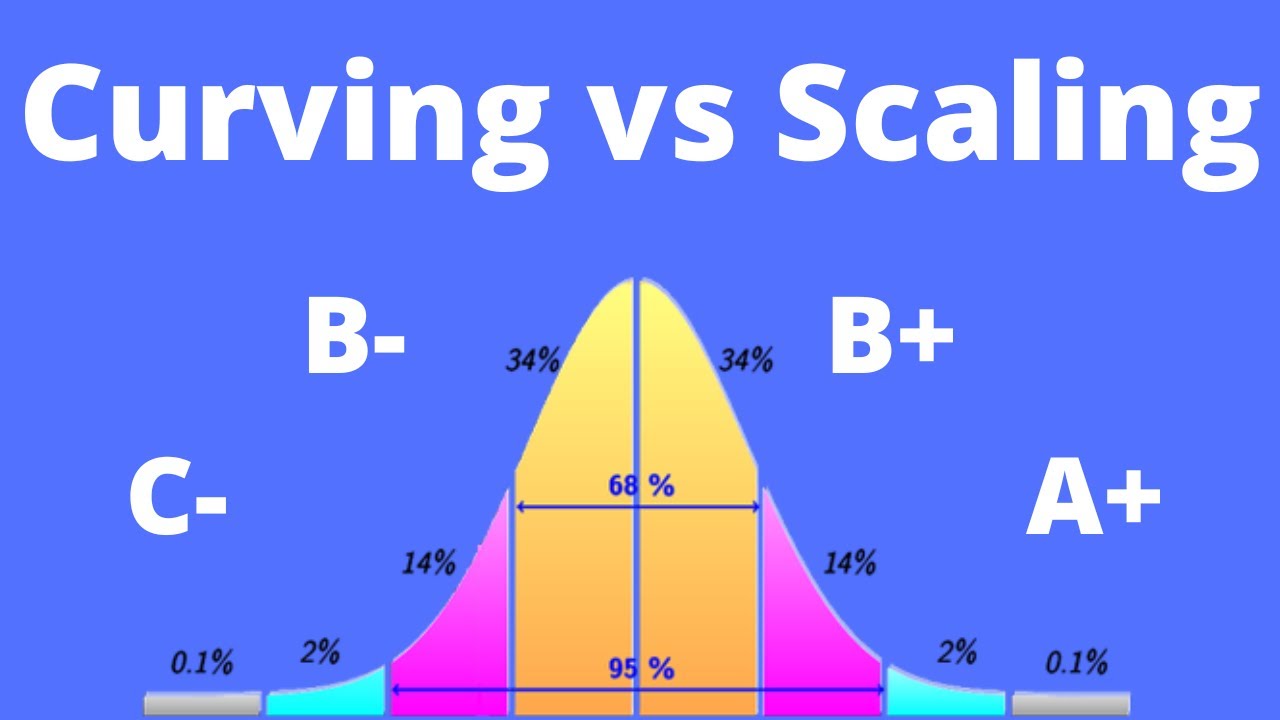
What is the curving graph called. The order of the letters is not. One simple kind of transformation involves shifting the entire graph of a function up, down, right, or left. Typically, the curve raises the average grade and individual student scores.

Grading with a curve means adjusting student scores after a test or assignment. The english idiom is the knee in the curve. this doesn't have much, if anything, to do with mathematics, however. What is the correct term to use for the top/bottom point?

Downward curve:a curve that turns in the downward direction is called a downward curve since it is curved. Line (curve) a geometrical concept, of which an exact and at the same time quite general definition presents considerable difficulties and is carried out differently in. In a graph, each edge can be named by the two letters of the associated vertices.

It is also known as a concave upward or convex downward curve. Top 10 colleges in indiana. Intuitively, a curve may be thought of as the trace left by a moving point.

The clearest indicators to check these answers with are (1) points where a function crosses zero compared to where another function has zero. This allows us to see all of the. Loosely speaking, the word curve is often used to.

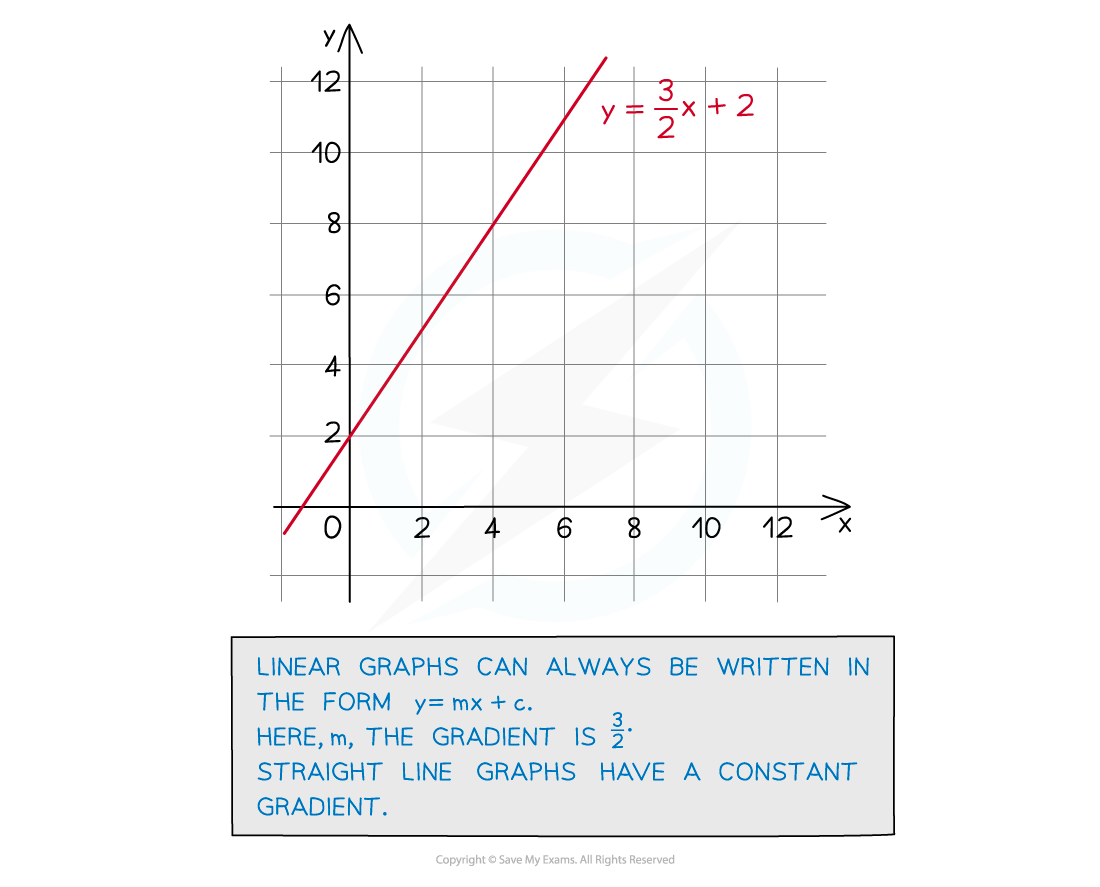
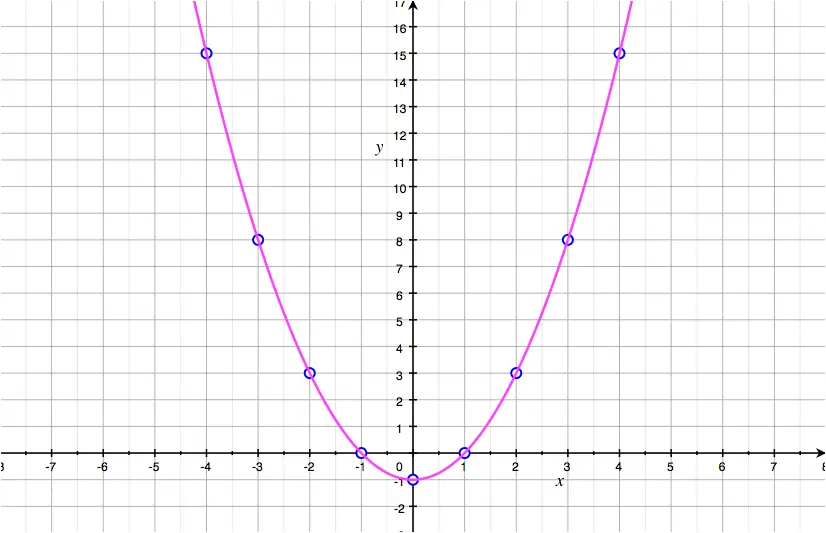
The simplest shift is a vertical shift, moving the graph up. Mathematics (including geometry, statistics, and applied mathematics ), physics, engineering,. The graph of a curve is given by $\{(a, f(a)):

A simple curving line can have a top peak and a bottom valley. In my native tongue, we call it a toppunkt, literally meaning. The point where the curve and the tangent meet is called the point of.

And the range of the curve is $\{f(a): Explore math with our beautiful, free online graphing calculator. In mathematics, a curve (also called a curved line in older texts) is an object similar to a line, but that does not have to be straight.

The [curved] line is […] the first species of quantity, which has only one dime… A function \(f(x)\) can be illustrated by its curve on an \(xy\) grid. I'm looking for a proper word or words that would describe a function that would start at zero, gradually climb toward one and then abruptly curve into the infinity.

This is the definition that appeared more than 2000 years ago in euclid's elements: It is continuous and smooth, without any sharp turns. This is a list of wikipedia articles about curves used in different fields: